
The interior angles of a shape are the angles inside it. So, for example, each of the exterior angles of a hexagon are 360/6 = 60°. Therefore if you have a regular polygon (in other words, where all the sides are the same length and all the angles are the same), each of the exterior angles will have size 360 ÷ the number of sides. because if you put them all together they form the angle all the way round a point: All of the exterior angles of a polygon add up to 360°.

The exterior angles of a hexagon are shown:Ī polygon is a shape with straight sides. The exterior angles of a shape are the angles you get if you extend the sides. Therefore the total angle sum of the quadrilateral is 360 degrees. Each triangle has an angle sum of 180 degrees. Now that we know the sum of the angles in a triangle, we can work out the sum of the angles in a quadrilateral.įor any quadrilateral, we can draw a diagonal line to divide it into two triangles. So the three angles in the triangle must add up to 180 degrees.

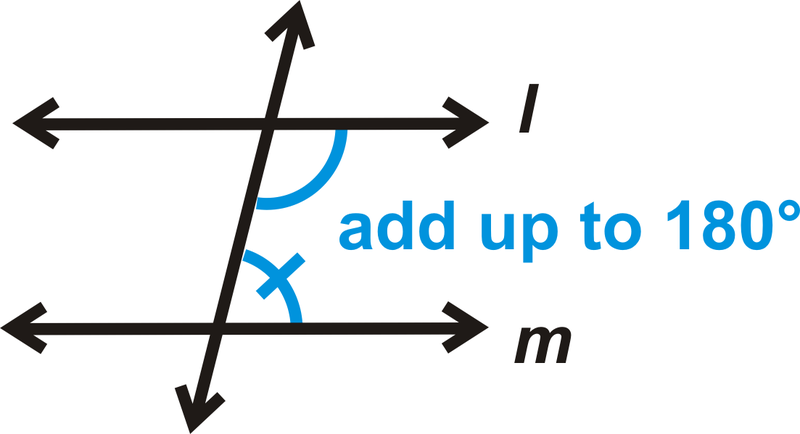
We know that x, y and z together add up to 180 degrees, because these together is just the angle around the straight line. Also, the two angles labelled y are equal. Therefore the two angles labelled x are equal. Now, we know that alternate angles are equal. If we have a triangle, you can always draw two parallel lines like this: Using some of the above results, we can prove that the sum of the three angles inside any triangle always add up to 180 degrees. These add up to 180 degrees (e and c are also interior).Īny two angles that add up to 180 degrees are known as supplementary angles. (d and c, c and a, d and b, f and e, e and g, h and g, h and f are also adjacent).ĭ and f are interior angles. Alternate angles form a 'Z' shape and are sometimes called 'Z angles'.Ī and b are adjacent angles. (h and d, f and b, e and a are also corresponding).ĭ and e are alternate angles. (b and c, e and h, f and g are also vertically opposite). Lines AB and CD are parallel to one another (hence the » on the lines).Ī and d are known as vertically opposite angles.


 0 kommentar(er)
0 kommentar(er)
